A jet is a condense and robust representation of a local grey value distribution. It is based on a Gabor wavelet transform, which is a convolution with a family of complex Gabor wavelets having the shape of plane waves restricted by a Gaussian envelope function. The wavelets are similar in the sense that they can all be generated from a mother wavelet by rotation and scaling. All complex coefficients of the transform taken at one image location form a jet.

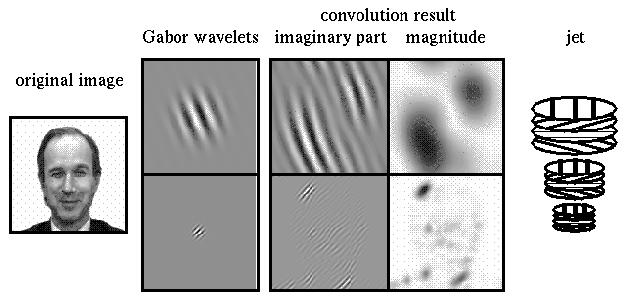
Since the Gabor kernels are wave-like, the coefficients vary with about the main frequency of the kernels, see imaginary part in the figure. This causes problem when comparing jets, because a small displacement may lead to very different coefficients. The magnitudes, however, vary slowly and can directly be used for comparison. One can also compensate for the fast coefficient variations and use them for an accurate displacement estimation.

Jets are robust with respect to illumination variations, scaling, translation, and distortion. Gabor wavelets are also a good model for the receptive fields of complex cells in primary visual cortex of primates.